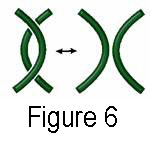
 The
second allows the adding or removal of two crossings. (see figure
6)
The
second allows the adding or removal of two crossings. (see figure
6)
 The
second allows the adding or removal of two crossings. (see figure
6)
The
second allows the adding or removal of two crossings. (see figure
6)
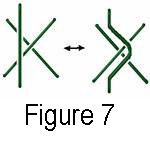 The
third allows the sliding of a strand of the knot from one side of a crossing
to the other side of the crossing. (see figure 7)
The
third allows the sliding of a strand of the knot from one side of a crossing
to the other side of the crossing. (see figure 7)
It can clearly be seen that these moves maintain equivalence between knots. The Reidemeister moves do in fact completely characterize knots. That is, if the Reidemeister moves are applied to one knot a finite number of times to get another knot, then the two knots are equivalent. This is proved in Gerhard Burde and Heiner Zeischang's book entitled Knots, and I will not show it here.[2]
Next Example: Tricolorability





